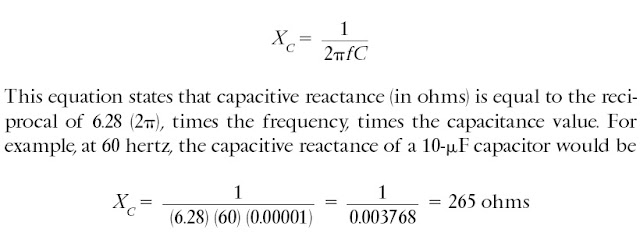
The Capacitors, like inductors, have a feature of frequency-dependent opposition to AC current flow called capacitive reactance (XC). When we apply AC voltage to a capacitor, it will charge and discharge in an effort to maintain a constant level of voltage. When frequency of the applied voltage to a capacitor is increased, capacitor’s opposition to AC current flow decreases. This is the main reason as to the amount of energy capable of being stored in the capacitor’s electrostatic field (it`s capacitance value) stays constant, but at the same moment the time period of the applied AC voltage decreases. it becomes pretty easy for the capacitor to fully absorb the charge of each half-cycle, as the AC time period decreases. In other point of view, it would require 10 times the capacity for a capacitor to charge for 10 milliseconds than it would for one millisecond. Therefore, the capacitive reactance decreases just when the frequency of an applied-voltage increases. Below is the equation for finding value capacitive reactance (Xc)
Note here that the value of capacitive reactance decreases as the level of frequency increases. After having examining the previous example of reactance, it should become clear that if an electrical circuit contained a combination of these 3 things that are resistors, capacitors, and inductors, the overall impedance of the circuit would be frequency dependent also.
Calculating the impedance of a resistive/reactive circuit is not easier as by simply adding reactance and resistance values together. Accurate impedance demands compensation for the phase difference between voltage and current. There are higher-level geometric equations used for calculating impedance for your personal interests and goals, you might never need to use them in future.









0 comments:
Post a Comment